Electric Field due to Multiple Point Charges

INTRODUCTION:

Electric field is a space surrounding electric charge in form of vector. It is induced by charge in the space prove by Coulomb’s law. Electric field contains electrical energy with energy density proportional to the square of the field intensity. The electric field is to charge as gravitational acceleration is to mass and force density is to volume.

In space, electric field also can be induced by more than one electrical charge. Derived from first Coulomb’s law and properties of superposition of electric charge, we can calculate the total electric field due to multiple charges.

Coulomb's law is absolutely fundamental; of course, it is consider a natural electrical phenomenon in physics. However, it is just as important in understanding and interpreting many kinds of chemical phenomena. For example, an atom is, in one respect, nothing other than a collection of electrical charges, positively charged protons, and negatively charged electrons. Columbic forces generated for electric field exist among these particles. For example, a fundamental problem involved in a study of the atomic nucleus is explaining how the enormous electrostatic force of repulsion among protons is overcome in such a way as to produce a stable body.

THE ELECTRIC FIELD:

In accordance with Coulomb's law, any charge Q produces a force field around itself, which is called the electric field. If this charge is immovable, the electric field is called electrostatic field. This field can be measured by a small test charge q fixed at any point at distance from the charge Q. According to coulomb's lawthe force on the test charge is directly proportional to its charge, so the ratio of this force to the value of the test charge does not depend upon the test charge q and is the unique characteristics of charge Q. This ratio is called the electric field intensity, , or just electric field, defined as the following vector

Thus the electric field is equal to the electric force per unit charge placed in this field. The unit of the electric field is newton per coulomb

The other unit of the electric field, frequently used, is volt per meter. We will show further that these units are the same.

Using coulomb's law we get the vector of the electric field produced by a point charge Q

with magnitude

Now we can see that this field does not depend upon the test charge q and depends only on the charge producing this field and the distance where it is measured.

The vector of this electric field is directed from the charge Q for positive charge and toward the charge for negative charge. This is shown in the figure 1 at an arbitrary point P

Figure 1: The electric field from the charge Q

Any electric field can be defined graphically by means of the electric field lines, as shown below

Figure 2: Electric field lines

The electric field lines are drawn as curves so that the tangent line to the curve at arbitrary point P is directed along the vector of the electric field at this point, and the density of lines is directly proportional to the magnitude of the electric field

where N is the number of lines crossing a small area A oriented normally to the electric field with the center at the point P, and s is an insignificant arbitrary scale parameter the same for all points.

Taking s = 1 we can rewrite the above formula in form

where the sign "" means numerical equality without taking units into account

The electric field with constant everywhere in both the magnitude and the direction is called a uniform electric field. The electric field lines of uniform field are shown below

Figure 2: Electric field lines

According to above formula the uniform electric field has a constant density of the electric field lines.

The electric field from a point charge is not uniform. Here the electric field lines are directed radially as shown below for positive (Q>0) and negative (Q<0)>

Figure 3: The electric field from a point charge is not uniform

Applying formulas for magnitude of electric and lines density, we get the density of field lines

Thus the electric field of a point charge has radial symmetry. Using , we get the total number of electric field lines for the electric field of a point charge

We got very important result for the point charge, that the total number of electric field lines is defined only by the value of the charge producing this electric field.

Electric Field due to Multiple Point Charges:

Now consider the more complex case, when the electric field is produced by two point charges Q₁ and Q_2, located at points defined by vectors and respectively, where we want to find the net electric field . We can readily get the electric fields and produced by charges Q₁ and Q₂ taken separately by using coulomb's law:

Figure 3: Electric field due to multiple point

Figure 4: Electric field due to multiple point

First, Find the force of

Second, Find the force of

The net electric field is equal to the vector sum of individual fields

Putting the above formulas gives

The vector can be readily determined graphically by parallelogram rule, which states that the vector is defined by the diagonal of the parallelogram with sides and . This is shown in the diagram below

The above equation is a mathematical notation of for two charges. In general the electric field due to multiple point charges states that the net electric field produced at any point by a system on n charges is equal to the vector sum of all individual fields produced by each charge at this point

general equestion

where is position vector of point P where the electric field is defined with respect to charge

The electric field due to multiple point charges seems to be evident. Nevertheless it cannot be derived from any fundamentals of Physics. Like the Coulomb's law, it is an experimental fact.

EXAMPLE:

Example 1,

Find the electric field, produced by a ring of radius R uniformly charged by charge Q, on the axis of the ring at a distance from its center

Figure 5: Electric field due to multiple point

Subdivide the ring into n pairs of diametrically opposite small portions each of charge , so that these portions can be considered as point charges. Then the electric fields produced by the two different portions of the pair at a point P are given respectively by:

From electric field due to multiple point charges we find that the resultant field produced by one portion is given by

Thus is directed along the axis of the ring.

Any other pair of opposite portions produces an electric field equal in magnitude and direction to . So, according to the electric field due to multiple point charges, the net electric field is given by

The above example gives a powerful algorithm for the calculation of an electric field of any charged object with arbitrary form and charge distribution. All we should do for this purpose is subdivide the object into n small charged portions and apply electric field due to multiple point chargesusing numerical integration over the volume of the object by a computer. The higher the number n the more accurate is the value of the electric field.

Example 2,

Two point charges with c and c are located in free space at (1,3,-1) and (-2,1,-2), respectively, in a Cartesian coordinate system. Find the electric field 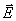at (3,1,-2)

solution

The electric field 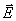with (free space) is given by

The vectors , and are given by

Hence,

RELATED TOPIC:

1. Vector
2. Electric force
3. Coulomb's law

CONCLUSION:

From our study, we have understand the concept of coulomb’s law, properties of electric charge and how to use them to generate equation to find the total electric field due to multiple charges. We also find that electric field play major part in understanding electrostatic and also electromagnetic.